Wird der Punkt \(P(1|2|3)\) an der Ebene \(E\) gespiegelt, so ergibt sich der Punkt \(Q(7|2|11)\).
Bestimmen Sie eine Gleichung von \(E\) in Koordinatenform.
(3 BE)
Lösung zu Teilaufgabe a
![Punkte P und Q, Ebene E, Mittelpunkt M der Strecke [PQ], Verbindungsvektor der Punkte P und Q](/images/stories/B2022_PT_A_G2/B2022_PT_A_G2_a.png)
Planskizze (optional): Der Verbindungsvektor \(\overrightarrow{PQ}\) ist zur Ebene \(E\) senkrecht. Damit ist jeder Vektor \(\textcolor{#0087c1}{k \cdot \overrightarrow{PQ}}\) mit \(k \in \mathbb R\) ein Normalenvektor der Ebene \(\textcolor{#cc071e}{E}\).
Der Mittelpunkt \(\textcolor{#cc071e}{M}\) der Strecke \([PQ]\) ist Fixpunkt der Spiegelung von \(P\) an der Ebene \(E\) bzw. Lotfußpunkt des Lotes von \(P\) auf \(E\) und liegt in der Ebene \(\textcolor{#cc071e}{E}\).
Ebenengleichung in Normalenform (vgl. Merkhilfe)
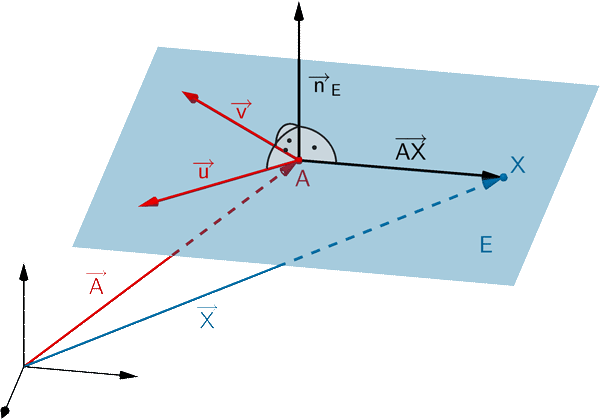
Jede Ebene lässt sich durch eine Gleichung in Normalenform beschreiben. Ist \(A\) ein beliebiger Aufpunkt der Ebene \(E\) und \(\overrightarrow{n}_{E}\) ein Normalenvektor von \(E\), so erfüllt jeder Punkt \(X\) der Ebene \(E\) folgende Gleichungen:
Normalenform in Vektordarstellung
\[E \colon \overrightarrow{n}_{E} \circ (\overrightarrow{X} - \overrightarrow{A}) = 0\]
Normalenform in Koordinatendarstellung
\[E \colon n_{1}x_{1} + n_{2}x_{2} + n_{3}x_{3} + n_{0} = 0\]
mit \(n_{0} = -(\overrightarrow{n}_{E} \circ \overrightarrow{A}) = - \: n_{1}a_{1} - n_{2}a_{2} - n_{3}a_{3}\)
\(n_{1}\), \(n_{2}\) und \(n_{3}\): Koordinaten eines Normalenvektors \(\overrightarrow{n}_{E}\)
Verbindungsvektor \(\overrightarrow{PQ}\) berechnen:
\(P(1|2|3)\), \(Q(7|2|11)\)
\[\overrightarrow{PQ} = \overrightarrow{Q} - \overrightarrow{P} = \begin{pmatrix} 7 \\ 2 \\ 11 \end{pmatrix} - \begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix} = \begin{pmatrix} 6 \\ 0 \\ 8 \end{pmatrix} = 2 \cdot \begin{pmatrix} 3 \\ 0 \\ 4 \end{pmatrix}\]
Somit ist \(\textcolor{#0087c1}{\overrightarrow{n} = \begin{pmatrix} 3 \\ 0 \\ 4 \end{pmatrix}}\) ein Normalenvektor der Ebene \(E\).
Ortsvektor des Mittelpunkts \(\textcolor{#cc071e}{M}\) der Strecke \([PQ]\) berechnen:
Mittelpunkt einer Strecke
Für den Ortsvektor \(\overrightarrow{M}\) des Mittelpunkts \(M\) einer Strecke \([AB]\) gilt:
\[\overrightarrow{M} = \frac{1}{2} \left( \overrightarrow{A} + \overrightarrow{B} \right)\]
\[\textcolor{#cc071e}{\overrightarrow{M}} = \frac{1}{2} \cdot (\overrightarrow{P} + \overrightarrow{Q}) = \frac{1}{2} \cdot \left[\begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix} + \begin{pmatrix} 7 \\ 2 \\ 11 \end{pmatrix}\right] = \textcolor{#cc071e}{\begin{pmatrix} 4 \\ 2 \\ 7 \end{pmatrix}}\]
Oder durch Vektoraddition:
![Bestimmung des Ortsvektors des Mittelpunkts M der Strecke [PQ] durch Vektoraddition](/images/stories/B2022_PT_A_G2/B2022_PT_A_G2_a_2.png)
\[\textcolor{#cc071e}{\overrightarrow{M}} = \overrightarrow{P} + \frac{1}{2} \cdot \overrightarrow{PQ} = \begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix} + \frac{1}{2} \cdot \begin{pmatrix} 6 \\ 0 \\ 8 \end{pmatrix} = \textcolor{#cc071e}{\begin{pmatrix} 4 \\ 2 \\ 7 \end{pmatrix}}\]
1. Möglichkeit: Ansatz mit der Normalenform in Vektordarstellung
Ebenengleichung in Normalenform (vgl. Merkhilfe)
Jede Ebene lässt sich durch eine Gleichung in Normalenform beschreiben. Ist \(A\) ein beliebiger Aufpunkt der Ebene \(E\) und \(\overrightarrow{n}_{E}\) ein Normalenvektor von \(E\), so erfüllt jeder Punkt \(X\) der Ebene \(E\) folgende Gleichungen:
Normalenform in Vektordarstellung
\[E \colon \overrightarrow{n}_{E} \circ (\overrightarrow{X} - \overrightarrow{A}) = 0\]

Normalenform in Koordinatendarstellung
\[E \colon n_{1}x_{1} + n_{2}x_{2} + n_{3}x_{3} + n_{0} = 0\]
mit \(n_{0} = -(\overrightarrow{n}_{E} \circ \overrightarrow{A}) = - \: n_{1}a_{1} - n_{2}a_{2} - n_{3}a_{3}\)
\(n_{1}\), \(n_{2}\) und \(n_{3}\): Koordinaten eines Normalenvektors \(\overrightarrow{n}_{E}\)
\[\begin{align*}E \colon &\;\textcolor{#0087c1}{\overrightarrow{n}} \circ (\overrightarrow{X} - \textcolor{#cc071e}{\overrightarrow{M})} = 0 \\[0.8em] E \colon &\textcolor{#0087c1}{\begin{pmatrix} 3 \\ 0 \\ 4 \end{pmatrix}} \circ \left[ \overrightarrow{X} - \textcolor{#cc071e}{\begin{pmatrix} 4 \\ 2 \\ 7 \end{pmatrix}} \right] = 0 \\[0.8em] &\,\textcolor{#0087c1}{3} \cdot (x_{1} - \textcolor{#cc071e}{4}) + \textcolor{#0087c1}{0} \cdot (x_{2} - \textcolor{#cc071e}{2}) + \textcolor{#0087c1}{4} \cdot (x_{3} - \textcolor{#cc071e}{7}) = 0 \\[0.8em] E \colon\, &3x_{1} -12 + 4x_{3} - 28 = 0 \\[0.8em] E \colon\, &3x_{1} + 4x_{3} - 40 = 0 \end{align*}\]
2. Möglichkeit: Ansatz mit der Normalenform in Koordinatendarstellung
Ebenengleichung in Normalenform (vgl. Merkhilfe)
Jede Ebene lässt sich durch eine Gleichung in Normalenform beschreiben. Ist \(A\) ein beliebiger Aufpunkt der Ebene \(E\) und \(\overrightarrow{n}_{E}\) ein Normalenvektor von \(E\), so erfüllt jeder Punkt \(X\) der Ebene \(E\) folgende Gleichungen:
Normalenform in Vektordarstellung
\[E \colon \overrightarrow{n}_{E} \circ (\overrightarrow{X} - \overrightarrow{A}) = 0\]

Normalenform in Koordinatendarstellung
\[E \colon n_{1}x_{1} + n_{2}x_{2} + n_{3}x_{3} + n_{0} = 0\]
mit \(n_{0} = -(\overrightarrow{n}_{E} \circ \overrightarrow{A}) = - \: n_{1}a_{1} - n_{2}a_{2} - n_{3}a_{3}\)
\(n_{1}\), \(n_{2}\) und \(n_{3}\): Koordinaten eines Normalenvektors \(\overrightarrow{n}_{E}\)
\(\textcolor{#0087c1}{\overrightarrow{n} = \begin{pmatrix} 3 \\ 0 \\ 4 \end{pmatrix}}\); \(\textcolor{#cc071e}{M(4|2|7)}\)
\[E \colon n_1x_1 + n_2x_2 + n_3x_3 + n_0 = 0\]
\[E \colon \textcolor{#0087c1}{3} \cdot x_1 + \textcolor{#0087c1}{0} \cdot x_2 + \textcolor{#0087c1}{4} \cdot x_3 + n_0 = 0\]
\[E \colon 3x_1 + 4x_3 + n_0 = 0\]
\[\begin{align*}\textcolor{#cc071e}{M} \in E \colon 3 \cdot \textcolor{#cc071e}{4} + 4 \cdot \textcolor{#cc071e}{7} + n_0 &= 0 \\[0.8em] 12 + 28 + n_0 &= 0 \\[0.8em] 40 + n_0 &= 0 &&| -40 \\[0.8em] n_0 &= -40\end{align*}\]
\[\Rightarrow \enspace E \colon 3x_1 + 4x_3 - 40 = 0\]


