Betrachtet werden die in \(\mathbb R\) definierten Funktionen \(f\) und \(F\), wobei \(F\) eine Stammfunktion von \(f\) ist. Abbildung 1 zeigt den Graphen \(G_F\) von \(F\).
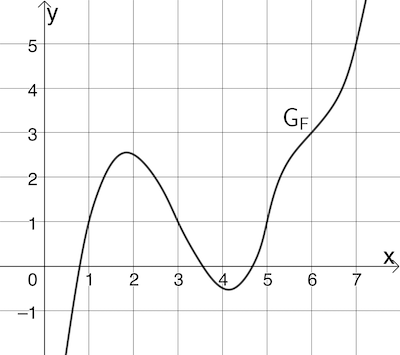Abb. 1
Bestimmen Sie den Wert des Integrals \(\displaystyle \int_1^7 f(x)dx\).
(2 BE)
Lösung zu Teilaufgabe 2a
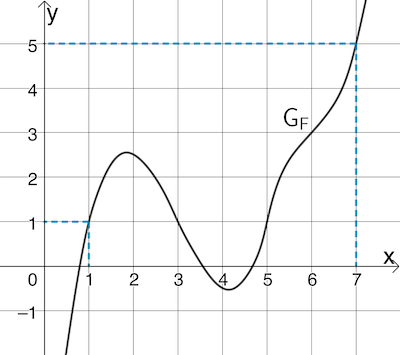Abb. 1
Mithilfe von Abbildung 1 ergibt sich:
Berechnung bestimmter Integrale
\[\int_{a}^{b} f(x)\,dx = [F(x)]_{a}^{b} = F(b) - F(a)\]
Dabei ist \(F\) eine beliebige Stammfunktion zu \(f\).
(vgl. Merkhilfe)
Eigenschaften des bestimmten Integrals - Integrationsregeln
Identische Integrationsgrenzen:
\[\int_{a}^{a} f(x)\,dx = 0\]
Faktorregel:
\(\displaystyle \int_{a}^{b} c \cdot f(x)\,dx = c \cdot \int_{a}^{b} f(x)\,dx\) mit \(c \in \mathbb R\)
Summenregel:
\[\int_{a}^{b} \left[f(x) \pm g(x) \right] dx = \int_{a}^{b}f(x)\,dx \pm \int_{a}^{b}g(x)\,dx\]
Vertauschungsregel:
\[\int_{a}^{b}f(x)\,dx = -\int_{b}^{a}f(x)\,dx\]
Zerlegung in Teilintervalle:
\(\displaystyle \int_{a}^{b}f(x)\,dx = \int_{a}^{c}f(x)\,dx + \int_{c}^{b}f(x)\,dx\) mit \(a \leq c \leq b\)
\[\int_1^7 f(x)dx = \textcolor{#0087c1}{F(7)} - \textcolor{#0087c1}{F(1)} = \textcolor{#0087c1}{5} - \textcolor{#0087c1}{1} = 4\]
